

Please note : I am a Harvard grad, SAT/ACT perfect scorer and full-time private tutor in Colorado Springs, Colorado, with over 20 years and 20,000 hours of professional teaching, coaching and tutoring experience. For more helpful information, check out my ACT Action Plan as well as my free e-book, Master the ACT by Brian McElroy. You should also check out my list of helpful ACT math TI calculator programs.
Google "ACT Math Formulas" and you get a grab-bag of subpar results, including an old PDF from 1996 and two popular but glaringly incomplete lists of ACT Math Formulas. The fact is that the ACT Math section has many more required formulas and concepts than the ones you can find easily online.
Don't despair--I'm here to help fill the gaps.
1) Law of Sines (in the past it was provided, but you have to memorize as of April 2021). (A / sin A) = (B / sin B) = (C / sin C) example 1 / example 2 / example 3
2) Law of Cosines (memorize also, just to be safe--the ACT usually provides it): c 2 = a 2 + b 2 - 2abcosC example 1 / example 2 / example 3
3) Formula for an Ellipse, and determining the Foci of an Ellipse (this was required on the April 2018 ACT #51 as well as the April 2021 ACT #59) Full Equation / Equation when Centered at 0,0
4) Products, Sums and Determinants of Matrices (welcome to the Matrix!). How to perform matrix calculations on your TI-83 Plus or above calculator / Augmented Matrices / Example Matrix Question
5) Sum of the First n terms of an Arithmetic Sequence and a Geometric Sequence
6) Nth term of an Arithmetic Sequence and Nth term of a Geometric Sequence
7) Translating logarithms (logs) to exponents and vice versa / the product, quotient and power rules of logarithms
8) Horizontal and Slant Asymptotes
9) Synthetic Division and Binomial Division (2010 June ACT #60)
10) The Complex Number Plane (2016 December ACT #57)
And hey, while we're at it, let's try for a full list of EVERY math formula and concept that shows up on the ACT (see below). If you feel that I've left anything out, then please let me know at mcelroy@post.harvard.edu.
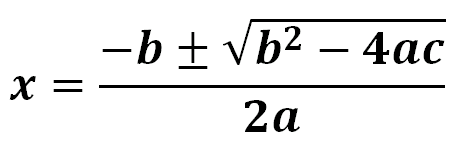
11) The Quadratic Equation (see below)
12) Percentage = (Part/Whole) and Percent Change = (Difference/Original) x 100
13) The Circle Proportionality Formula (Area of "Slice"/Area of Whole = Arc Length/Circumference = Measure of Inner Angle/360)
14) The Formula for a Line (slope intercept y=mx+b format, standard form Ax + By = C, and point-slope format: y-y1 = m(x-x1), and the slope equation (y2-y1) / (x2-x1) ).
15) All 3 Quadratic Identities (unfactored to factored form)
(x 2 -y 2 )=(x+y)(x-y)
x 2 +2xy+y 2 =(x+y) 2
x 2 -2xy+y 2 =(x-y) 2
16) The Third Side Rule for Triangles (a-b) < c < (a+b) if c represents the “third side” and b and a represent the lengths of the other two sides.
17) Direct and Indirect Proportion ( (a1/b1)=(a2/b2) and (a1a2 = b1b2)
18) Average = (Total / Number of things)
19) Probability = (Desired Possibilities / Total Possibilities).
20) Surface Area of a Cube = 6s 2
21) Distance = Rate x Time
22) Weighted Averages
23) Simultaneous Equations / Substitution
24) Functions
25) Imaginary numbers (i) and the iterations of i. Binomial addition involving constants and i by combining like terms (adding and subtracting complex numbers)
26) Multiplying by the complex conjugate of the denominator to simplify complex number fractions
27) Completing the square
28) Sin x = Cos (90-x)
29) Concept: the vertex of a parabola is located at the midpoint of its x-intercepts, or using the formula -b/2a
30) The vertex (h,k) form of a parabola: a(x-h) 2 + k and equation of a hyperbola (image) / (#52 December 2019 ACT Form C03)
31) Area of a non-right triangle = 1/2 ab sin C
32) Concept: when an upward projectile reaches its highest point, its velocity is zero.
33) Concept: when an upward projectile lands, its height is zero.
34) Concept: the sides of similar triangles all have the same respective proportions.
35) Concept: in a system of linear equations, there is no solution if the slopes of the two lines are the same (parallel) and the y-intercept is different. Conversely, there are infinitely many solutions is the slopes of the two lines are the same and the y-intercept is also the same.
36) Concept: to find the intersections of two lines, set them equal to one another
37) Concept: the “zeroes” or "roots" of a function are the x-coordinates where it crosses the x-axis (and where the y value outputs zero).
38) Concept: the degree measure of an arc formed by an angle with its vertex on a circle is double the measure of the angle, or equal the measure of the circle if the vertex is on the center of the circle.
39) Concept: the value of a function is undefined when the denominator is equal to zero.
40) Concept: t he proportion of distance that you travel along the one leg of a triangle is equal to the proportion of distance that you travel along both other legs.
41) The equation of a circle with center (h,k) and radius r: (x-h) 2 + (y-k) 2 = r 2
42) The Polynomial Remainder Theorem
43) Domain (all possible x inputs), Range (all possible y outputs), and Interquartile Range (2023 April Form F11 #19)
44) Manipulating Absolute Value Inequalities
45) Negative and Fractional Exponents
46) Rules of Exponents: "Same Root" Tricks (multiplication = add the exponents, division = subtract the exponents, taking to a power = multiply the exponents). "Same Exponent" Trick (perform the operation on the base and keep the exponent the same for multiplication and division operations). Also know how to calculate fractional powers.
47) Parallel Lines and Transversals
48) Positive and Negative Associations in Graphs
49) π radians = 180 degrees
50) Permutations and Combinations
51) Area of a Trapezoid = [(a+b)/2]h]
52) Standard Deviation (more on SD). Standard Deviation appeared on #59 of the December 2016 ACT. You don't need to know the equation—only the concept—but you can use your calculator to solve (link).
53) Area of a Circle = π r 2
54) Circumference of a Circle = 2πr
55) Area of a Triangle = (base)(height)/2
56) Volume of a Rectangular solid = (length)(width)(height)
57) Volume of a Cylinder = π r 2 h
58) Volume of a Cone = (1/3) π r 2 h
59) Number of degrees in an n-sided shape: (n-2)(180)
60) Adding Vectors in Component Form (tests #2 and #3 from the new book)
61) Concept: a polynomial of Nth degree has at most N-1 changes in direction.
62) Euler's Formula: Vertices + Faces - Edges = 2 / example
63) Polar Coordinates: x = r cos( θ ) and y = r sin( θ ) / 2015 April ACT #59
64) sin 2 x + cos 2 x = 1 (#46 2018 June ACT)
65) Stem and Leaf Plots (#21 June 2023 ACT Form F12)
That’s all you need to know as far as formulas and concepts!
YOU SHOULD ALSO KNOW THE DEFINITIONS OF THE FOLLOWING TERMS:
-PEMDAS AND THE ORDER OF OPERATIONS. If you don’t know what I’m talking about here, talk to your math teacher, pronto! Just a reminder…Parentheses, Exponents, Multiplication, Division, Addition, Subtraction. Also remember that a TI-83 (perfectly legal on this test) automatically performs PEMDAS so long as you enter the expression correctly.
- MEAN, MEDIAN, MODE. Mean is the same as average. Median is the number in the middle after rearranging from low to high. In the case that the list has no true middle because it has an even number of terms, find the average of the middle two. So the median of the list < 1 1 5 5 >is (1+5)/2 which equals 3. MODE is quite simply the number that appears the MOST. Multiple modes are possible if there is a tie for greatest frequency: the example I just listed, for example, has two modes, 1 and 5. To calculate the median of an odd number of terms, simply add 1 and divide by 2. To calculate the median of an even number of terms n, take the average of the (n/2) term and the following term.
-INTEGERS. Integers are whole numbers, including zero and negative whole numbers. Think of them as hash marks on the number line. (For those who don’t know what hash marks are, picture the while yardage markings on the grass of a football field.) Don’t forget that zero is an integer and that negative whole numbers are integers too. Remember that -3 is less than -2, not the other way around (sounds simple but is a common mistake. If I fooled you initially with that one, think of “greater than” as “further to the right” on a number line, and “less than” as “further to the left.”
-PRIME NUMBERS. Prime numbers are positive integers that are only divisible by themselves and the number 1. Be able to list all the primes you between 1 and 50…remember that 1 is not a prime and there are no negative primes. By the way, 51 is not prime…that question actually showed up on a recent SAT. 17 x 3 = 51. What, you forgot your times tables for 17? ;)
Also, be able to use a factor tree and find all the factors of a number and perform a “prime factorization” of a number (this means you find a series of prime numbers that multiplies together to equal that number). The prime factorization of 18, for example, is 3 x 3 x 2.
-PYTHAGOREAN TRIPLES. These are particular types of Right Triangles that just happen to have exact integers as sides. The SAT loves to use them, so know them by heart and save yourself the trouble of calculating all those roots. Here are the ones they use:
3/4/5, 5/12/13, 6/8/10, 7/24/25, 8/15/17
Please note that Pythagorean Triples are not the same as 45/45/90 and 30/60/90 triangles, which are provided for you at the beginning of each Math section.)
-“Y LESS THAN X: for example, “x - 7” is the correct mathematical translation of “7 less than x.” Be careful because many students will write this as “7 - x”, which is incorrect.
-THE WORD “OF.” (“of” always means multiply.)
-DIGITS. Digits are to numbers what letters are to words. There are only 10 possible digits, 0 through 9.
-MULTIPLES. The MULTIPLES of x are the ANSWERS I get when I MULTIPLY x by another INTEGER. For example the multiples of 5 are 5,10,15,20 etc. as well as 0 (a multiple of everything because anything times zero is zero) as well as -5, -10, -15 and other NEGATIVE MULTIPLES.
-FACTORS. The factors of x are the answers I get when I divide x by another integer. For example the factors of 60 are 30, 20,15,12,10,6,5,4,3,2,1, as well as -5,-6,-10 etc.
-REMAINDER. Remainder is the whole number that’s left over after division. For example 8/3 equals 2 remainder 2. Remainder is particularly helpful on pattern and sequence problems.
-CONSECUTIVE INTEGERS. Consecutive integers are integers in order from least to greatest, for example 1,2,3. The ACT may also ask for consecutive even or odd integers. For example -6,-4,-2, 0, 2, 4 etc (yes zero is even) or 1, 3, 5 etc.
-SUM. Sum means the result of addition. The sum of 3 and 5 is 8. I know, duh, but you’d be surprised how many students will say “15” if they are not paying close attention.
-DIFFERENCE. Difference is the result of subtraction.
-PRODUCT. The result of multiplication. Do not confuse with sum!
-ODD AND EVEN NUMBERS. Even numbers are all the integers divisible by 2, and odd numbers are all the other integers.
-POSITIVE and NEGATIVE NUMBERS. Be aware that if the problem asks for “a negative number,” that does not necessarily mean a negative INTEGER. -1.5 will do just fine. Zero is neither negative nor positive. Be aware of strange tricks with negatives, and that negatives taken to EVEN powers are positive and that negatives taken to ODD powers are negative.
-GEOMETRY and TRIGONOMETRY. You’re going to have to remember basic geometrical concepts (180 degrees in a line, 360 degrees in a quadrilateral, 360 degrees in a circle, all radii of a circle are equal 180 degrees in a triangle, rules of parallel lines and transversals, trapezoids have two parallel sides, vertical angles are congruent, perpendicular lines have slopes that are negative reciprocals of each other.). Also be familiar with the formulas for Sine (Opposite/Hypotenuse), Cosine (Adjacent/Hypotenuse) and Tangent (Opposite/Adjacent) in right triangles, which can be remembered with the acronym "SOHCAHTOA". Also be familiar with the inverses of these trigonometric functions and the reciprocals of these trigonometric functions -- the reciprocal of sine is cosecant (csc), the reciprocal of cosine is secant (sec), and the reciprocal of tangent is cotangent (cot).
-COINCIDENT LINES are just a fancy term for "two of the the exact same line" (2023 June ACT #48)
That being said, the fewer formulas you need to remember, the more you can focus on technique, and good technique is the true key to an excellent ACT score. I don’t teach my students unnecessary formulas because I can teach them to find the answers using a more logical approach to the problem.
This isn’t math class, where you have to show your work or use “proper” technique. This is the ACT, where the only thing that matters is that you get the correct answer as quickly as possible. So you can get away with shortcuts galore. This is why the best ACT math tutors focus on problem recognition, technique and logic more than they focus on pure memorization.
In other words, these formulas are a great tool and do allow for shortcuts, but you should also focus on logical and conceptual understanding skills, and taking plenty of practice ACTs to hone your skills. Studying a formula sheet is no substitute for putting in the hard work by taking at least 8-10 full ACT practice tests, and then reviewing the results properly, waiting a few weeks, and then re-trying questions from scratch (in other words, without seeing your previous work, a.k.a. "blind review") until you can answer them correctly.
For more information you can read my ACT Action Plan.